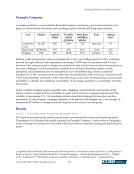
Emerging Practices for Capital Adequacy © Copyright 2003, CCRO. All rights reserved. 46 7.3.3 Monte Carlo Simulation - Combined Simulation Approach The third method of combining market, credit, and operative risk components is to use Monte Carlo simulation. Two methods can be used to combine the impact of market, credit, and operative risk into one distribution. One method consists of jointly simulating all relevant risk factors so that the combined impact of market, credit, and operative risk is measured in every scenario. For example, price changes used in deriving market risk are also used to determine the exposure used in credit risk. Here the inputs are driven off a consistent price propagation process. In addition to this, the simulation output for market, credit, and operative risk is aggregated, creating a single joint probability distribution depicting the outcomes of the value of a firm’s deals and current business. Another method uses numerical integration techniques instead of joint simulation. It is described as the convolution of the underlying distributions for market, credit, and operative risks, using appropriate correlations. Convolution is the mathematical operation that groups each distribution with the next. Convolution requires an assumption as to the form of the copula (the joint probability density function for the set of outcomes from multiple random variables, with each variable’s outcome expressed in terms of its marginal cumulative density function). To evaluate this convolution without joint simulation, we must break up the problem into a series of two- distribution convolutions, the result of each subsequently convolved with the next input distribution, i.e. a “pair-wise roll-up” (e.g., aggregating market and credit risk and then aggregating the resulting distribution with operative risk). From the final distribution, total economic capital can be determined by measuring the distance between the mean and a specified confidence level. Both approaches require extensive computational and modeling resources. However, the numerical integration method generalizes to as many distributions as are desired with only linear cost in computational intensity, as opposed to multi-dimensional calculations or simulations, which are exponential in computational resources. Both methods would provide an in depth analysis and insight into potential future outcomes. Compared with the two other combined capital methodologies, this causes a company to show a higher level of capital adequacy, all things being equal. Although the methodology requires greater resources and investment, the benefit is a reduced economic capital measure and thus a higher level of capital adequacy. In summary, there should be a built-in incentive for companies to pursue methodologies of greater sophistication. 7.4 Summary of Selected Economic Capital Methodology After choosing a particular methodology for combining market, credit, and operative risk, a company should be prepared to discuss the assumptions used in arriving at a particular number for total economic capital. Table 6 provides a summary of the three methodologies proposed and can be a guideline for that discussion. Note that modern portfolio theory and simulation, because of their sophistication, may yield lower economic capital requirements.